The Platonic solids are unique in many ways. As you might imagine, much has already been written about the Platonic solids. Mathematicians view them are the building blocks of geometry. Physical scientists are interested in them because they can be found all throughout the basic structures of the universe. Spiritual teachers see them as a part of sacred geometry. We are interested in them because they are all nested within Metatron’s Cube.
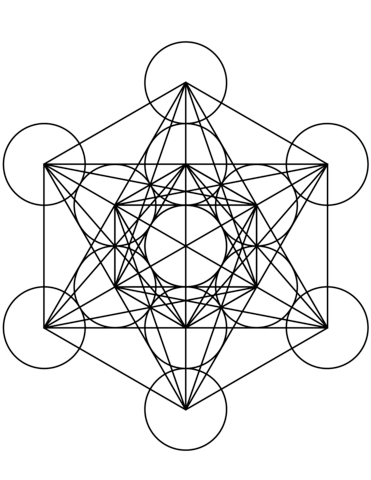
The five Platonic solids are all contained within this two-dimensional image of Metatron's Cube that is shown above. When you attempt to isolate the Platonic solids, they are, of course, also shown in two-dimension, however, they are drawn in three-point perspective. The Platonic solids are all three-dimensional figures.
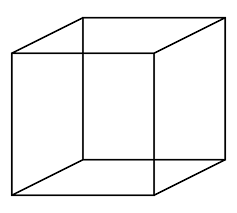
The next Platonic solid that we are interested in is the hexahedron. The hexahedron has six sides. A regular hexahedron has six faces, and each face is an equilateral square. Equilateral squares have all four sides the same length. See the image below.
Metatron’s cube has at least two regular hexahedrons in it. Let’s begin with tracing the larger hexahedron from the two-dimensional image of Metatron’s cube.
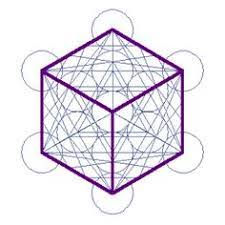
When you find the smaller hexahedron you will notice that the two of them together form a tesseract. The tesseract is not one of the Platonic solids.

Click on the video below which focuses on the larger hexahedron to see the animation of it turning into a three- dimensional shape.
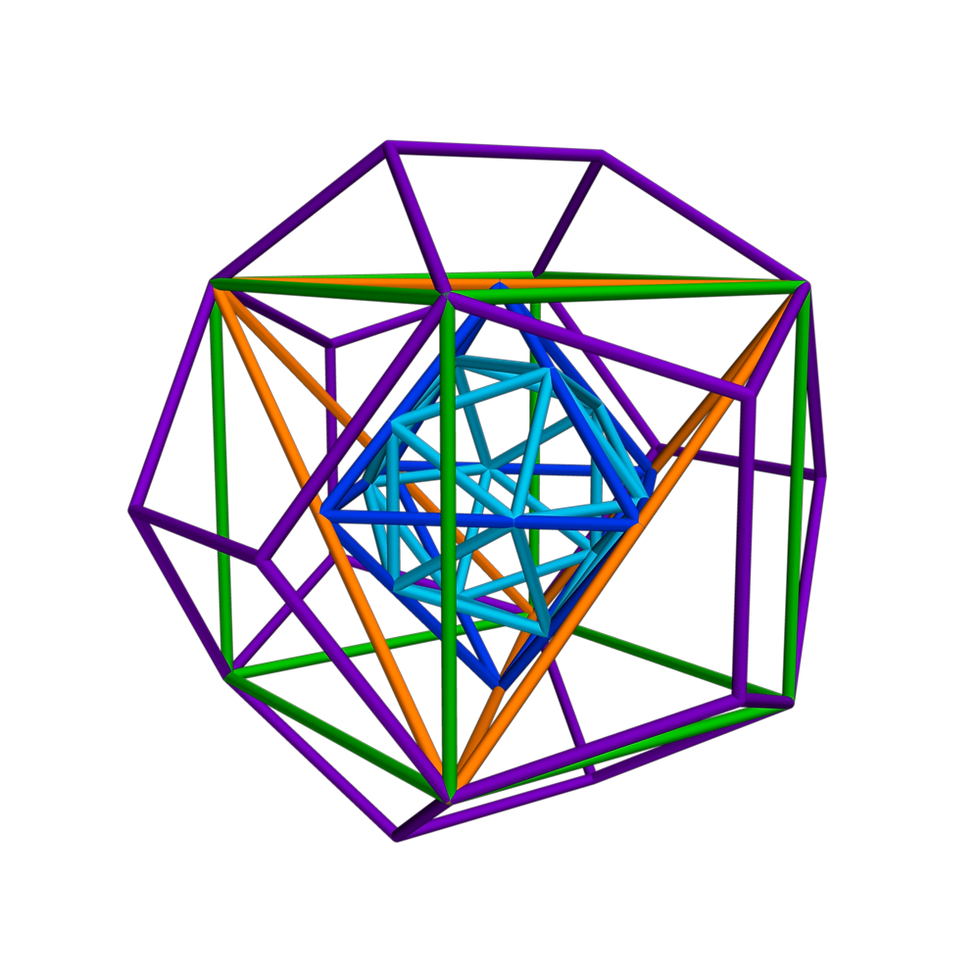
The three-dimensional model of Metatron’s cube that we are working to build is shown below. This model is only a partial model since it only shows one example of each of the Platonic solids. It is important because it shows the nesting of the Platonic solids within each other. This nesting allows them to freely rotate within Metatron’s cube. It is the rotation of the Platonic solids that generates the energy within Metatron’s cube.
Comments